five regular polyhedra

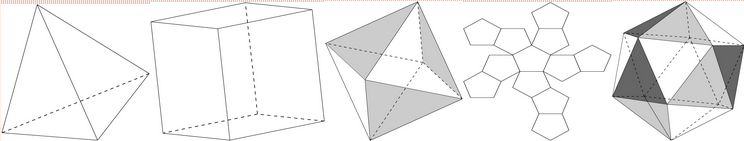
A regular polyhedron, also known as a Platonic solid, refers to a three-dimensional shape where all its faces are regular polygons of the same size and all its vertices have the same number of edges meeting at them. There are only five such regular polyhedra, as shown in the following figures:

The proof is quite simple：   Let's consider a fixed vertex N. Around this vertex, there are a regular b-sided polygons (as in the case of the dodecahedron, where a = 3 and b = 5)，   The measure of each angle in a regular polygon with b sides is ，   Since each angle in a regular b-sided polygon measures , the sum of all angles around vertex N is 。

Upon cutting along the edges, we observe that these angles can be spread out around point N on a plane without overlapping (since we assume the regular polyhedra are convex). 。

Simplifying the expression, we have (a - 2)(b - 2) < 4.

Therefore, there are only five possible combinations for a and b:

a = 3, b = 3 (Tetrahedron)

a = 3, b = 4 (Cube)

a = 4, b = 3 (Octahedron)

a = 3, b = 5 (Dodecahedron)

a = 5, b = 3 (Icosahedron)

Furthermore, all five combinations are actually valid.

In ancient Greek philosophy, the four basic elements of fire, earth, air, and water were represented by the tetrahedron, cube, octahedron, and icosahedron, respectively. The shape of the entire universe was believed to be a dodecahedron. Due to their significance in the philosophy of Plato, these five regular polyhedra are also known as Platonic solids.